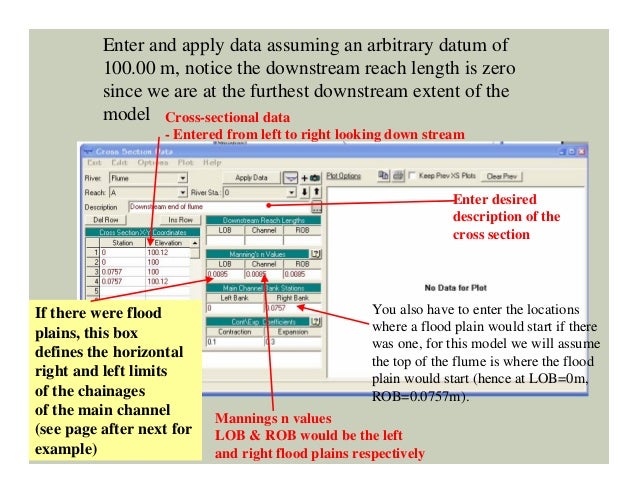
treeserious.blogg.se - Hec ras culvert tutorial

HEC RAS CULVERT TUTORIAL FULL

HEC RAS CULVERT TUTORIAL FULL

Once the computations begin, RAS will use the full dynamic form of the Momentum equation and Sf will be recomputed using an average Sf between 2 cross sections. Yes, So and Sf are equated in your example, but that is only for Manning's equation and for establishing the initial water surface elevation at the downstream end. Boundary is solved first, to establish a starting water surface. Solving the boundary condition is completely independant of the Cons of Momentum equation. But this is one of the reasons we like to have the boundary condition far away from your area of interest, so that any errors you do introduce because of your assumption, will have diminished prior to reaching your area of interest. If you use your bed slope, then you are claiming uniform flow, which is most likely not the case (in a natural stream), but is usually close enough for a boudnary condition. RAS is actually solving the Manning's equation discretely at the downstream cross section using the slope you provide. It might be wise to move the downstream boundary downstream a little further. Here, you can see with the sensitivity study, the three profiles (with three different downstream boundary energy slopes) converge at the bridge. Caution…for you steep slope, make sure you do not force the results to critical depth by selecting a slope that is too steep. If it is below your area of interest than the boundary is far enough downstream. Plot both results on the profile plot and see where the solutions for both energy slopes converge. Bracket a range of realistic energy slopes and then run both the high and low value. For example, if I’m working on a bridge hydraulics study, I try to get the downstream boundary at least 5 cross section widths downstream of the bridge. Move the downstream boundary location as far away from my area of interest as possible.

To manage the error, I do a couple of things: But, if we can manage the error associated with using normal depth, it can serve as a very convenient tool for estimating the downstream boundary condition. Besides the fact that you have to more-or-less guess at an energy slope, natural rivers and streams rarely-if ever exhibit uniform flow conditions. Regardless of the method you use to get an energy slope, there will be some error associated with what you use. Measure the distance between the first two contour lines that cross the your stream.

Use a USGS Quad map or other contour file or terrain model of the reach downstream of your modeled reach.

This will better approximate the energy slope at the downstream boundary, but you can run into problems if your reach ends on a horizontal or adverse slope.

Measure the bed slope of the last two cross sections at the downstream boundary.

The pop-up window gives you a dy/dx term, which is the slope. You can do this by holding the ctrl key and drawing a line to represent the average slope as shown above.

Measure the average bed slope of your stream in the profile plot.

There are a few ways you can assume an energy slope for your downstream boundary: Well, without computing, this is impossible to come by, and in order to compute, you have to assume an energy slope. To be true to Manning’s equation, the correct answer is the discrete energy slope at the downstream cross section.

The most common question I get about using the Normal Depth option is what slope to use. as the flow changes, so will the downstream boundary depth). This method’s popularity stems from its ease of use and it’s semi dynamic properties (i.e. This allow you to provide an energy slope, and then RAS will automatically back-calculate the depth using Manning’s Equation. You make an assumption that your river flows under normal flow (uniform flow) conditions at the downstream boundary of your model. Probably the most commonly used downstream boundary condition in both steady and unsteady RAS is the Normal Depth assumption.